Why Is It Very Much Important To Have a Good Command Of Determinants And Matrices?

The determinants and matrices in linear algebra are considered to be one of the most important chapters and it is very much important for the kids to be familiar with different kinds of rules in the whole process so that they can calculate things perfectly. The determinants will always be calculated for the square matrices and if the determinant of the matrix will be zero then it will be referred to as singular determinant and if it is one then it will be referred to as unimodular. For this particular system of equations, it is very much important for the kids to have a unique solution and the determinant of the matrix must be non-singular so that value can be non-0.
The matrices are considered to be the order to rectangle area of numbers that can be perfectly utilised in terms of expressing the linear equations this particular matrix will be having rows and columns so that mathematical operations can be perfectly performed without any kind of problem. It is also very much important to indulge in addition, subtraction, multiplication of the matrix and several other kinds of things so that there is no hassle throughout the process.
The kids need to be very much clear about different kinds of concepts like symmetric matrix, diagonal matrix, upper triangular matrix, lower triangular matrix, identity matrix, zero matrix and many other kinds of things that will always provide them with the best possible way of finding out the answers. Hence, being clear about all the above-mentioned procedures along with multiplicative Inverse is very much important for the kids so that they can score well in their mathematics examination and further it is important for the kids to enrol themselves on platforms like Cuemath so that all the doubts are very easily cleared by the best quality teachers and there is no problem in the exams.
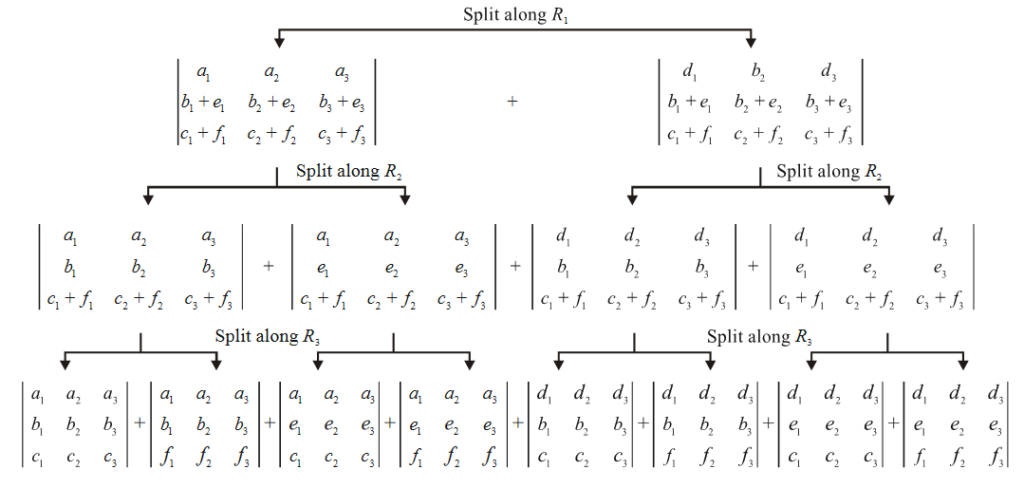
The determinant can be defined in several kinds of ways for the square matrix and the first and most simple way to formulate it is to take into account the top row elements and the corresponding minors then the students need to take the first element of the top row and multiplied by its minor so that they can subtract the product of the second element from the minor very easily. They need to continue this particular process till they can achieve the identity matrix without any kind of problem.
The second method to find out the best possible determinants is explained as follows:
The second way is to define the determinants is to define the whole concept in terms of columns by the matrix by expressing the N into N matrix in the term of column vectors. The column vectors will be found with the help of implementation of different kinds of formulas and where the scalar is will be denoted by B vector of size X will be denoted by V identity matrix of size X will be denoted by I.
Basic properties of the determinants:
- If I N is the identity matrix of the order and into in the determinant of I will be is equal to 1.
- If the matrix is MT is the transport of matrix M the determinant of matrix MT will be determinant of matrix M.
- If matrix M -1 is the inverse of Matrix M then the determinant of M -1 is equal to the determinant of M raised to power -1.
- If two square matrices M and end will be having the same size then the determinant will be determinant of M into a determinant of N.
- If the matrix is a triangular one then the determinant will be equal to the product of diagonal elements.
- In the cases of the determinant of a matrix is zero then elements of Matrix will also be zero.
- The determinants also help in following different kinds of properties like all zero property, factor property, triangle property, reflection property, switching property and various other kinds of things.






